MP 113

Premise
Nomenclature inconsistencies and conflicts can best be resolved through a detailed understanding of the origin and development of terms. The goal of this project is to investigate the origins and use as well as prior and current definitions of resolution and resolving power in order to make informed recommendations on the controversial and in some cases conflicting terminology.
Current Definitions
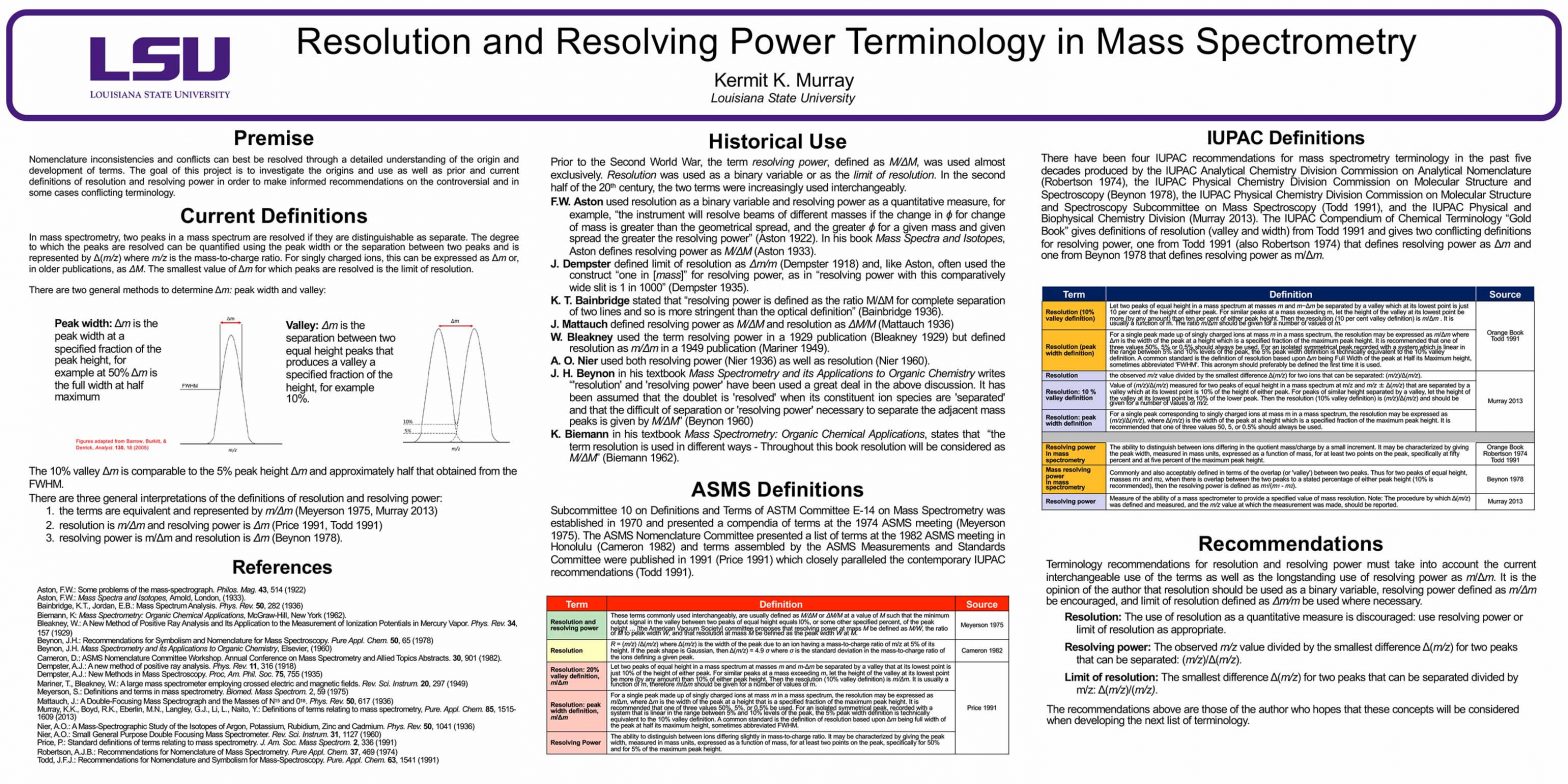
In mass spectrometry, two peaks in a mass spectrum are resolved if they are distinguishable as separate. The degree to which the peaks are resolved can be quantified using the peak width or the separation between two peaks and is represented by Δ(m/z) where m/z is the mass-to-charge ratio. For singly charged ions, this can be expressed as Δm or, in older publications, as ΔM. The smallest value of Δm for which peaks are resolved is the limit of resolution.
There are two general methods to determine Δm: peak width and valley:
Peak width: Δm is the peak width at a specified fraction of the peak height, for example at 50% Δm is the full width at half maximum
Valley: Δm is the separation between two equal height peaks that produces a valley a specified fraction of the height, for example 10%.
The 10% valley Δm is comparable to the 5% peak height Δm and approximately half that obtained from the FWHM.
There are three general interpretations of the definitions of resolution and resolving power:
• the terms are equivalent and represented by m/Δm (Meyerson 1975, Murray 2013)
• resolution is m/Δm and resolving power is Δm (Price 1991, Todd 1991)
• resolving power is m/Δm and resolution is Δm (Beynon 1978).
Historical Use
Prior to the Second World War, the term resolving power, defined as M/ΔM, was used almost exclusively. Resolution was used as a binary variable or as the limit of resolution. In the second half of the 20th century, the two terms were increasingly used interchangeably.
F.W. Aston used resolution as a binary variable and resolving power as a quantitative measure, for example, “the instrument will resolve beams of different masses if the change in ϕ for change of mass is greater than the geometrical spread, and the greater ϕ for a given mass and given spread the greater the resolving power” (Aston 1922). In his book Mass Spectra and Isotopes, Aston defines resolving power as M/ΔM (Aston 1933).
J. Dempster defined limit of resolution as Δm/m (Dempster 1918) and, like Aston, often used the construct “one in [mass]” for resolving power, as in “resolving power with this comparatively wide slit is 1 in 1000” (Dempster 1935).
K. T. Bainbridge stated that “resolving power is defined as the ratio M/ΔM for complete separation of two lines and so is more stringent than the optical definition” (Bainbridge 1936).
J. Mattauch defined resolving power as M/ΔM and resolution as ΔM/M (Mattauch 1936)
W. Bleakney used the term resolving power in a 1929 publication (Bleakney 1929) but defined resolution as m/Δm in a 1949 publication (Mariner 1949).
A. O. Nier used both resolving power (Nier 1936) as well as resolution (Nier 1960).
J. H. Beynon in his textbook Mass Spectrometry and its Applications to Organic Chemistry writes “’resolution’ and ‘resolving power’ have been used a great deal in the above discussion. It has been assumed that the doublet is ‘resolved’ when its constituent ion species are ‘separated’ and that the difficult of separation or ‘resolving power’ necessary to separate the adjacent mass peaks is given by M/ΔM” (Beynon 1960)
K. Biemann in his textbook Mass Spectrometry: Organic Chemical Applications, states that “the term resolution is used in different ways – Throughout this book resolution will be considered as M/ΔM” (Biemann 1962).
ASMS Definitions
Subcommittee 10 on Definitions and Terms of ASTM Committee E-14 on Mass Spectrometry was established in 1970 and presented a compendia of terms at the 1974 ASMS meeting (Meyerson 1975). The ASMS Nomenclature Committee presented a list of terms at the 1982 ASMS meeting in Honolulu (Cameron 1982) and terms assembled by the ASMS Measurements and Standards Committee were published in 1991 (Price 1991) which closely paralleled the contemporary IUPAC recommendations (Todd 1991).
| Term | Definition | Source |
| Resolution and resolving power | These terms commonly used interchangeably, are usually defined as M/ΔM or ΔM/M at a value of M such that the minimum output signal in the valley between two peaks of equal height equals l0%, or some other specified percent, of the peak height … [the American Vacuum Society] committee proposes that resolving power at mass M be defined as M/W, the ratio of M to peak width W, and that resolution at mass M be defined as the peak width W at M. | Meyerson 1975 |
| Resolution | R = (m/z) /Δ(m/z) where Δ(m/z) is the width of the peak due to an ion having a mass-to-charge ratio of m/z at 5% of its height. If the peak shape is Gaussian, then Δ(m/z) = 4.9 σ where σ is the standard deviation in the mass-to-charge ratio of the ions defining a given peak. | Cameron 1982 |
| Resolution: 20% valley definition, m/Δm | Let two peaks of equal height in a mass spectrum at masses m and m-Δm be separated by a valley that at its lowest point is just 10% of the height of either peak. For similar peaks at a mass exceeding m, let the height of the valley at its lowest point be more (by any amount) than 10% of either peak height. Then the resolution (10% valley definition) is m/Δm. It is usually a function of m, therefore m/Δm should be given for a number of values of m. | Price 1991 |
| Resolution: peak width definition, m/Δm | For a single peak made up of singly charged ions at mass m in a mass spectrum, the resolution may be expressed as m/Δm, where Δm is the width of the peak at a height that is a specified fraction of the maximum peak height. It is recommended that one of three values 50%, 5%, or 0.5% be used. For an isolated symmetrical peak, recorded with a system that is linear in the range between 5% and 10% levels of the peak, the 5% peak width definition is technically equivalent to the 10% valley definition. A common standard is the definition of resolution based upon Δm being full width of the peak at half its maximum height, sometimes abbreviated FWHM. | |
| Resolving Power | The ability to distinguish between ions differing slightly in mass-to-charge ratio. It may be characterized by giving the peak width, measured in mass units, expressed as a function of mass, for at least two points on the peak, specifically for 50% and for 5% of the maximum peak height. |
IUPAC Definitions
There have been four IUPAC recommendations for mass spectrometry terminology in the past five decades produced by the IUPAC Analytical Chemistry Division Commission on Analytical Nomenclature (Robertson 1974), the IUPAC Physical Chemistry Division Commission on Molecular Structure and Spectroscopy (Beynon 1978), the IUPAC Physical Chemistry Division Commission on Molecular Structure and Spectroscopy Subcommittee on Mass Spectroscopy (Todd 1991), and the IUPAC Physical and Biophysical Chemistry Division (Murray 2013). The IUPAC Compendium of Chemical Terminology “Gold Book” gives definitions of resolution (valley and width) from Todd 1991 and gives two conflicting definitions for resolving power, one from Todd 1991 (also Robertson 1974) that defines resolving power as Δm and one from Beynon 1978 that defines resolving power as m/Δm.
| Term | Definition | Source |
| Resolution (10% valley definition) | Let two peaks of equal height in a mass spectrum at masses m and m−Δm be separated by a valley which at its lowest point is just 10 per cent of the height of either peak. For similar peaks at a mass exceeding m, let the height of the valley at its lowest point be more (by any amount) than ten per cent of either peak height. Then the resolution (10 per cent valley definition) is m/Δm . It is usually a function of m. The ratio m/Δm should be given for a number of values of m. | Orange Book Todd 1991 |
| Resolution (peak width definition) | For a single peak made up of singly charged ions at mass m in a mass spectrum, the resolution may be expressed as m/Δm where Δm is the width of the peak at a height which is a specified fraction of the maximum peak height. It is recommended that one of three values 50%, 5% or 0.5% should always be used. For an isolated symmetrical peak recorded with a system which is linear in the range between 5% and 10% levels of the peak, the 5% peak width definition is technically equivalent to the 10% valley definition. A common standard is the definition of resolution based upon Δm being Full Width of the peak at Half its Maximum height, sometimes abbreviated ‘FWHM’. This acronym should preferably be defined the first time it is used. | |
| Resolution | the observed m/z value divided by the smallest difference Δ(m/z) for two ions that can be separated: (m/z)/Δ(m/z). | Murray 2013 |
| Resolution: 10 % valley definition | Value of (m/z)/Δ(m/z) measured for two peaks of equal height in a mass spectrum at m/z and m/z ± Δ(m/z) that are separated by a valley which at its lowest point is 10% of the height of either peak. For peaks of similar height separated by a valley, let the height of the valley at its lowest point be 10% of the lower peak. Then the resolution (10% valley definition) is (m/z)/Δ(m/z) and should be given for a number of values of m/z. | |
| Resolution: peak width definition | For a single peak corresponding to singly charged ions at mass m in a mass spectrum, the resolution may be expressed as (m/z)/Δ(m/z), where Δ(m/z) is the width of the peak at a height which is a specified fraction of the maximum peak height. It is recommended that one of three values 50, 5, or 0.5% should always be used. | |
| Resolving power In mass spectrometry | The ability to distinguish between ions differing in the quotient mass/charge by a small increment. It may be characterized by giving the peak width, measured in mass units, expressed as a function of mass, for at least two points on the peak, specifically at fifty percent and at five percent of the maximum peak height. | Orange Book Robertson 1974 Todd 1991 |
| Mass resolving power In mass spectrometry | Commonly and also acceptably defined in terms of the overlap (or ‘valley’) between two peaks. Thus for two peaks of equal height, masses m1 and m2, when there is overlap between the two peaks to a stated percentage of either peak height (10% is recommended), then the resolving power is defined as m1/(m1 – m2). | Beynon 1978 |
| Resolving power | Measure of the ability of a mass spectrometer to provide a specified value of mass resolution. Note: The procedure by which Δ(m/z) was defined and measured, and the m/z value at which the measurement was made, should be reported. | Murray 2013 |
Recommendations
Terminology recommendations for resolution and resolving power must take into account the current interchangeable use of the terms as well as the longstanding use of resolving power as m/Δm. It is the opinion of the author that resolution should be used as a binary variable, resolving power defined as m/Δm be encouraged, and limit of resolution defined as Δm/m be used where necessary.
Resolution: The use of resolution as a quantitative measure is discouraged: use resolving power or limit of resolution as appropriate.
Resolving power: The observed m/z value divided by the smallest difference Δ(m/z) for two peaks that can be separated: (m/z)/Δ(m/z).
Limit of resolution: The smallest difference Δ(m/z) for two peaks that can be separated divided by m/z: Δ(m/z)/(m/z).
The recommendations above are those of the author who hopes that these concepts will be considered when developing the next list of terminology.
References
Aston, F.W.: Some problems of the mass-spectrograph. Philos. Mag. 43, 514 (1922)
Aston, F.W.: Mass Spectra and Isotopes, Arnold, London, (1933).
Bainbridge, K.T., Jordan, E.B.: Mass Spectrum Analysis. Phys. Rev. 50, 282 (1936)
Biemann, K: Mass Spectrometry: Organic Chemical Applications, McGraw-Hill, New York (1962).
Bleakney, W.: A New Method of Positive Ray Analysis and Its Application to the Measurement of Ionization Potentials in Mercury Vapor. Phys. Rev. 34, 157 (1929)
Beynon, J.H.: Recommendations for Symbolism and Nomenclature for Mass Spectroscopy. Pure Appl. Chem. 50, 65 (1978)
Beynon, J.H. Mass Spectrometry and its Applications to Organic Chemistry, Elsevier, (1960)
Cameron, D.: ASMS Nomenclature Committee Workshop. Annual Conference on Mass Spectrometry and Allied Topics Abstracts. 30, 901 (1982).
Dempster, A.J.: A new method of positive ray analysis. Phys. Rev. 11, 316 (1918)
Dempster, A.J.: New Methods in Mass Spectroscopy. Proc, Am. Phil. Soc. 75, 755 (1935)
Mariner, T., Bleakney, W.: A large mass spectrometer employing crossed electric and magnetic fields. Rev. Sci. Instrum. 20, 297 (1949)
Meyerson, S.: Definitions and terms in mass spectrometry. Biomed. Mass Spectrom. 2, 59 (1975)
Mattauch, J.: A Double-Focusing Mass Spectrograph and the Masses of N15 and 018. Phys. Rev. 50, 617 (1936)
Murray, K.K., Boyd, R.K., Eberlin, M.N., Langley, G.J., Li, L., Naito, Y.: Definitions of terms relating to mass spectrometry, Pure. Appl. Chem. 85, 1515-1609 (2013)
Nier, A.O.: A Mass-Spectrographic Study of the Isotopes of Argon, Potassium, Rubidium, Zinc and Cadmium. Phys. Rev. 50, 1041 (1936)
Nier, A.O.: Small General Purpose Double Focusing Mass Spectrometer. Rev. Sci. Instrum. 31, 1127 (1960)
Price, P.: Standard definitions of terms relating to mass spectrometry. J. Am. Soc. Mass Spectrom. 2, 336 (1991)
Robertson, A.J.B.: Recommendations for Nomenclature of Mass Spectrometry. Pure Appl. Chem. 37, 469 (1974)
Todd, J.F.J.: Recommendations for Nomenclature and Symbolism for Mass-Spectroscopy. Pure. Appl. Chem. 63, 1541 (1991)